

Appendix: Special Topics
This page offers more detail on several home theater design topics summarized in the previous theater build pages. Topics on this page include more information on Minimum and Maximum Field of View, Speaker Volume Estimation, and Riser Height Estimation. I worked through each of these topics myself with some help from the internet. If you find any mistakes, let me know, and send a comment.
Estimating the Field of View
Last Updated: 09/19/2024
The Field of View (FOV) calculation is straightforward but requires a little high-school trigonometry. Looking at Figure 1, we can treat the distance to the screen and half the screen's width as two sides of a right triangle. Using the arc tangent function, we can take the two sides and determine the angle from the center of the screen to the edge of the screen. The FOV is then twice this angle, as shown in the last equation.
Using the example distances of 12 ft. (144 in.) and half the screen width of 48", one can calculate the FOV using twice the arc tangent, 36.8 degrees.
Two organizations are typically sighted (by various websites) when looking for recommended field-of-view guidelines.
-
SMPTE recommends a minimum FOV of 30 degrees for movie theaters.
-
THX recommends an optimum FOV of 36 degrees for a movie theater (2.35:1) and a minimum viewing angle of 40 degrees for a 16:9 screen.
Note: There are variations in the information published by various individuals. Some of the information posted is very old. Figure 2 seems to be a good summary (see here for the original) but has not been confirmed (by the author) with the original specifications. (The author does not want to pay for the SMPTE EG 18, Design of Effective Cine Theaters.)

Figure 1. FOV Equations

Figure 2. SMPTE/THX Theater FOV Recommendations (hopefully)
How Close is too Close to the Screen?
Last Updated: 09/02/2024
How close one sits to the screen can be a personal preference and depends on several factors.
-
Sitting so close, one uncomfortably moves eyes around the screen too much to see the whole image.
-
Sitting so close that one can start to see the individual pixels.
The first is a personal preference, which seems to be partially accounted for in the THX and SMPTE recommendations. The second is due to the human eye's resolution, discussed below.
Minimum (separable) resolvable acuity—A person of regular sight, measured as 20/20 vision, can differentiate two objects that cast a visual angle of 1 minute (60 seconds or 0.017 degrees). (see Evaluation of Visual Acuity)
If one is sitting 12 feet from a 115-inch HD projector/screen (which has 1920 horizontal pixels), the field of view (FOV) is ~36.8 degrees. The angle of a single pixel is 0.019. Thus, individual pixels will likely not be detectable unless one has excellent eyesight, in which case pixels may be barely detectable. The equation used here is:
The pixel angle of a UHD projector/screen with a horizontal resolution of 3840 will be half. Even at a six-foot seating distance, a 4K 115" screen will have a pixel angle of ~0.017.

Figure 3. Your too close when you can see individual pixels
Speaker Volume at the Listening Position
Last Updated: 07/30/2025
Typical listening volume can be a personal preference. We need to define our near-maximum listening volume to determine whether the audio system can play loud enough. Some enthusiasts answer this question by ensuring their system can operate at full "reference volume." According to THX specifications, reference levels for home theater calibration are 85 dB SPL average and 105 dB peak SPL for any given individual full-range channel. The LFE channel is 115 dB peak. (Note: Typical audio calibration tools use 75dB reference signals and scales internally.)
For this room, since I generally listen to movies, TV, or music at an average volume of around 75dB (and this is with multiple speakers), I decided to relax the volume goal to 75-80dB average and 95-100dB peak.
So, how can one determine if the design meets this goal? Especially when we don't have the tools (or are too lazy) to do a detailed simulation of the room. One can get a reasonably good estimate from a few basic equations and some estimates of the room's gain/loss contribution.
Speaker Sensitivity—This specifies how loud a speaker is at a 1M distance. See Figure 4. However, there are a couple of caveats because different manufacturers may measure speaker sensitivity differently.
-
There are two ways manufacturers specify this. The original test method applies a 1-watt signal to the speaker and the audio level is measured 1-meter from the speaker. The more recent methodology is the same except to apply a 2.83-Volt signal to the speaker which for an 8Ω speaker is equivalent to 1-Watt, but if the speaker's nominal impedance is other than 8Ω then the input power is different, and the sensitivity specification needs to be adjusted.
-
The equations assume this specification does not include boundary reinforcement (or room gain). Room gain (aka room reinforcement) will reduce energy loss, and adjustments are needed to account for it.
Seating Distance—The distance the listener is sitting away from the speaker. If we know the listener's seating distance and speaker sensitivity, we can determine the expected volume at our seat.
Amplifier Gain—Normally, we power speakers with more than 1 watt, so this is the amplifier's gain beyond the reference. See equation 3 below.
Room Reinforcement—The equations below are based on an anechoic room (no reflections). In an actual room, reflections reduce sound loss. To determine this exact amount, detailed modeling is required. However, there are some published approximations, which I used to compensate for this. For a small room, the loss reduction is about 3-6 dB.

Figure 4. Picture showing speaker and distance to listener
Using the above, the basic equation for SPL at the listener's position is:
The loss is due to distance from the speaker. Assumes no room reinforcement and a single speaker.
Gain due to Amplifier:
The full equation becomes this:

Distance (ft) | Distance (m) | SPL Loss |
|---|---|---|
3.28 | 1 | 0 |
4 | 1.22 | 1.7 |
5 | 1.52 | 3.7 |
6 | 1.83 | 5.2 |
6.56 | 2 | 6 |
7 | 2.13 | 6.6 |
8 | 2.44 | 7.7 |
9 | 2.74 | 8.8 |
9.84 | 3 | 9.5 |
10 | 3.05 | 9.7 |
11 | 3.35 | 10.5 |
12 | 3.66 | 11.3 |
13 | 3.96 | 12 |
13.12 | 4 | 12 |
14 | 4.27 | 12.6 |
15 | 4.57 | 13.2 |
Amplifier Power (W) | Amplifier Gain (dB) (1W Reference) |
|---|---|
200 | 23.01 |
150 | 21.76 |
100 | 20 |
80 | 19.03 |
40 | 16.02 |
20 | 13.01 |
10 | 10 |
5 | 6.99 |
2 | 3.01 |
1 | 0 |
Table 1. Ideal SPL loss (left) and amplifier gain (right)
Using equation 4, even without knowing which speaker will be chosen, I could estimate the sound volume for this theater. We can use some typical numbers to give a general idea of what is possible.
-
A typical number for a bookshelf speaker's sensitivity is ~85dB.
-
A typical value for amplifier power is 100W per channel.
-
For this home theater, the listening distance is ~12ft or 3.66 meters.
Substituting these values into the equation results in:
Assuming no room reinforcement, the calculation results in an SPL of 94 dB. The estimated impact of room reinforcement is a 4-6 dB gain at the listening position, which results in a 98-100dB maximum SPL. Even though these design assumptions don't meet peak reference SPL specifications, reaching ~100dB is not quite full reference volume, but it is acceptably loud to me.
Table 1 shows SPL losses for a range of seating distances and amplifier gains, expressed in dB. These show the difference distance and amplifier power can make.
Some additional informative links on room audio volume considerations:
More on Speaker Sensitivity
Some clarification is needed regarding the sensitivity measurement. Using 1 Watt as the input power is convenient because it makes it easy to adjust the audio level based on the power supplied by an amplifier, as shown above. However, when using a fixed voltage, you must consider the power differences across various loads. The lower the impedance, the more power is delivered. To illustrate this, refer to the equations below. Equation #5 shows the basic power formula in terms of applied voltage and speaker impedance. Rearranging and solving for voltage (Equation 6), we see that to deliver 1 Watt to an 8Ω speaker, you need to apply 2.83V. Applying this 2.83V to a 4Ω speaker results in supplying 2 Watts (equation 8).
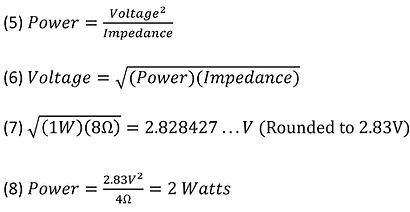
or a 4Ω speaker specified with a 2.83V input, the sensitivity is adjusted to find the 1-Watt sensitivity. Equation #3 can be used for this calculation, which shows that the sensitivity decreases by 3dB, since 10log(2) = 3. For example, a 4Ω speaker with a sensitivity of 85dB with a 2.83V test signal will have an 82dB sensitivity at 1-Watt input.
Even after doing these calculations, it is important to remember that the results are very approximate due to several factors. First, none of these equations accounts for room gain. Second, a manufacturer's speaker impedance specification is approximate and is rarely exactly 4 or 8 Ω.
Calculating the Riser Height
Last Updated: 09/02/2024
For a two-row home theater, the second row must be raised to give the screen a clear line of sight. Alternatively, I suppose the screen could be raised if one has room above it, but this usually results in uncomfortable sightlines. (The viewer has to look up.)
A simple approach to estimating the riser's height is treating the second-row sightline as part of two triangles. This is shown in Figure 5 with the purple dotted lines. One triangle's hypotenuse is from the back row viewer's eyes to the bottom of the screen, and the two sides of this triangle are the back row viewer's distance from the screen and his eye height above the bottom of the screen. The second similar triangle is from the front row viewer's height and distance from the screen. The riser height calculation uses the ratio of these two triangles to determine how high the back-row viewer's eyes need to be.
The picture in Figure 5 below is the derivation of the equations used to calculate the riser height. The result is Equation #4, which describes riser height as the ratio of the distance from the screen of each row times the difference in the height of each row (without the riser). The height of each row can be measured reclined or not. I went with not reclined for both rows.
Equation 4 can be simplified, assuming that the front row is halfway between the second row and the screen and that the seat/viewer height is roughly the same for both rows (HT1 and HE1 in Figure 5). The riser height is the difference between the height of the front row (top of the viewer's head) and the bottom of the screen.
For this home theater, HT1 (front row height) is ~42 in., and SF (bottom of screen) is ~30 in. Thus, the approximate riser height should have been ~12 in.

Figure 5. Riser height triangles and detailed equations to derive a formula to estimate back row height.
